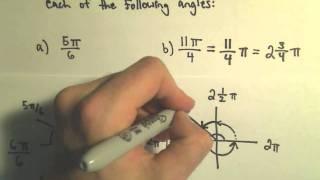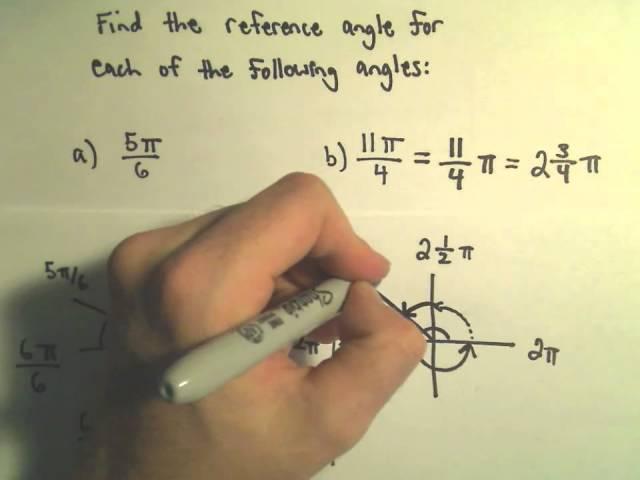
Reference Angle for an Angle, Ex 2 (Using Radians)

I love you from the moon back!! Thanks Patrick!

Omg thank you so much. the videos on my eBook website sucked at explaining this. now i get it thank you!

You just saved my life for my finals!!

Thank you very much. Your videos helped me a lot!

Amazing!! Thanks for this sir !! :)))))))))

You are the reason for my B in College Algebra last semester. Now in Trig you helping me out. Amazing work

Thanks for the help!!!!!!!!

you need to explain why your way to descriptive

We didnt even learn it this way. We have to convert the radians to degrees and find the reference angles that way. This wouldve been so much easier.

Thank you very much ! 

God bless bro 

Dude! You saved my butt on my math homework! Thanks!

hello guys, can someone please tell me how someone can find for example SINE40 without using calculator??? thanks

Okay I'm kind of still confused. Can you also find the reference angle by converting radians to degrees by multiplying the given number by 180/pi? For example if you're given 5pi/6 can't you just multiply this by 180/pi cross out the pi's and simplify to get your degrees and then take it from there or is that totally totally wrong?

Thank you so much!

i just convert to degrees then convert back to radians when im done

how do you find associated points patrickJMT

Thank you!

Pre Calc final tomorrow.... Wish me luck??

Patrick,
Nice work. However, I show my students that for an angle given in radians in the form m*pi/n, that the reference angle is merely pi/n. They still have to decide what quadrant the original angle is in to properly evaluate the appropriate trig function. At least for the major angles with denominators of 6, 4 and 3.

I'm really confused on the 11pi/4 one. Wouldnt you subtract 2pi instead of 3pi because 11pi/4 is in quad four?

why do you keep the reference angle positive when the actual angle is negative?

I never thought about putting it into a mix number. Professor, why you know teach us short cuts!

thank you !

Notice how it's always pi over the original denominator. You don't have to do any work

This was so nicely explained! I'm so glad I came across this channel ^_^ Thank you so much!

This helped me so much!!! This kind of thing was really stumping me! I can't thank you enough!

Im sorry but i didn't understand anything .... ik its my fault lol but fr trig is my ultimate enemy.

Thanks for this. My teacher has the worst teaching methods for math I've ever encountered and I have a test tomorrow. I might be able to do a little bit better now.

If you don't like dealing with radians in these kinds of problems it is easy to convert to degrees then going from there, if your answer needs to be in radians then convert back at the end.

Thanks

6pi- 5pi is NOT 1PI! ITS ONLY PI!

Bruh you really helped me with my unit test, thank you

i am still confused

Wow. It's so helpful! Thank you so much. Because of that, I SUBSCRIBED.

Super helpful video!

An easy way to figure out where it lies is to divide up pi (so each 180 degrees)up into sections by the denominator, and then "move" the number of sections required
for example: if its 3pi/4, divide the top and bottom part of the graph into 4 sections each (8 sections total) and then move 3 sections. Saves the guess work. If it was 11pi/4, you would move 11 sections around the graph

I'm even more confused than when I started.

great video! My teachers nor friends were able to explain this as clearly and easily as you did! thank you

what about what if the given is -13pi/3? isn't it going to be the reference angle itself?

Best one...

I saw this man already so bie

Life saver frfr

omg a few seconds from you and it just clicked in my mind, a few seconds from my prof and I'm already giving up, thank you for this!

I don't understand anything lol

thank uuuu smmmmm this helps a lot!

please let me know how
- pi/6 is equal to 5pi/6

My module for this lesson never seemed to clearly explain how to figure out which quadrant a radian is in, so thank you for this video! ^_^

his hand cover evthing becasue hes a lefty

this is really helpful, but i am not quite getting to grips with 15 angle (5pi/8)